Homogeneous trigonometric equations of degree 2. Homogeneous trigonometric equations: general solution scheme

Lesson topic: "Homogeneous trigonometric equations"

(10th grade)

Target: introduce the concept of homogeneous trigonometric equations of degree I and II; formulate and work out an algorithm for solving homogeneous trigonometric equations of degrees I and II; teach students to solve homogeneous trigonometric equations of degrees I and II; develop the ability to identify patterns and generalize; stimulate interest in the subject, develop a sense of solidarity and healthy competition.

Lesson type: lesson in the formation of new knowledge.

Form: work in groups.

Equipment: computer, multimedia installation

Lesson progress

Organizational moment

Greeting students, mobilizing attention.

In the lesson, a rating system for assessing knowledge (the teacher explains the system for assessing knowledge, filling out the assessment sheet by an independent expert selected by the teacher from among the students). The lesson is accompanied by a presentation. .

Updating basic knowledge.

Homework is checked and graded by an independent expert and consultants before class and a score sheet is completed.

The teacher summarizes the performance homework.

Teacher: We continue to study the topic “Trigonometric equations”. Today in the lesson we will introduce you to another type of trigonometric equations and methods for solving them, and therefore we will repeat what we have learned. When solving all types of trigonometric equations, they are reduced to solving the simplest trigonometric equations.

Individual homework done in groups is checked. Defense of the presentation “Solutions of the simplest trigonometric equations”

(The work of the group is assessed by an independent expert)

Motivation for learning.

Teacher: We have work to do to solve the crossword puzzle. Having solved it, we will find out the name of a new type of equations that we will learn to solve today in class.

Questions are projected onto the board. Students guess, and an independent expert enters the scores of the students who answer on the score sheet.

Having solved the crossword puzzle, the children will read the word “homogeneous”.

Assimilation of new knowledge.

Teacher: The topic of the lesson is “Homogeneous trigonometric equations.”

Let's write down the topic of the lesson in a notebook. Homogeneous trigonometric equations are of first and second degree.

Let us write down the definition of a homogeneous equation of the first degree. I show an example of solving this type of equation; you create an algorithm for solving a homogeneous trigonometric equation of the first degree.

Equation of the form A sinx + b cosx = 0 is called a homogeneous trigonometric equation of the first degree.

Let us consider the solution to the equation when the coefficients A And V are different from 0.

Example: sinx + cosx = 0

R dividing both sides of the equation term by cosx, we get

Attention! You can divide by 0 only if this expression does not turn to 0 anywhere. Let’s analyze. If the cosine is equal to 0, then the sine will also be equal to 0, given that the coefficients are different from 0, but we know that the sine and cosine go to zero at different points. Therefore, this operation can be performed when solving this type of equation.

Algorithm for solving a homogeneous trigonometric equation of the first degree: dividing both sides of the equation by cosx, cosx 0

Equation of the form A sin mx +b cos mx = 0 also called a homogeneous trigonometric equation of the first degree and also solve the division of both sides of the equation by the cosine mx.

Equation of the form a sin 2 x+b sinx cosx +c cos2x = 0 called homogeneous trigonometric equation second degree.

Example : sin 2 x + 2sinx cosx – 3cos 2 x = 0

Coefficient a is different from 0 and therefore, like the previous equation, cosx is not equal to 0, and therefore you can use the method of dividing both sides of the equation by cos 2 x.

We get tg 2 x + 2tgx – 3 = 0

We solve by introducing a new variable let tgx = a, then we get the equation

a 2 + 2a – 3 = 0

D = 4 – 4 (–3) = 16

a 1 = 1 a 2 = –3

Back to replacement

Answer:

If the coefficient a = 0, then the equation takes the form 2sinx cosx – 3cos2x = 0, we solve it by taking the common factor cosx out of brackets. If the coefficient c = 0, then the equation takes the form sin2x +2sinx cosx = 0, we solve it by taking the common factor sinx out of brackets. Algorithm for solving a homogeneous trigonometric equation of the first degree:

See if the equation contains the asin2 x term.

If the term asin2 x is contained in the equation (i.e. a 0), then the equation is solved by dividing both sides of the equation by cos2x and then introducing a new variable.

If the term asin2 x is not contained in the equation (i.e. a = 0), then the equation is solved by factorization: cosx is taken out of brackets. Homogeneous equations of the form a sin2m x + b sin mx cos mx + c cos2mx = 0 are solved in the same way

The algorithm for solving homogeneous trigonometric equations is written in the textbook on page 102.

Physical education minute

Formation of skills for solving homogeneous trigonometric equations

Opening problem books page 53

1st and 2nd groups decide No. 361-v

The 3rd and 4th groups decide No. 363-v

Show the solution on the board, explain, complement. An independent expert evaluates.

Solving examples from problem book No. 361-v
sinx – 3cosx = 0
we divide both sides of the equation by cosx 0, we get

No. 363-v
sin2x + sinxcosx – 2cos2x = 0
divide both sides of the equation by cos2x, we get tg2x + tanx – 2 = 0

solve by introducing a new variable
let tgx = a, then we get the equation
a2 + a – 2 = 0
D = 9
a1 = 1 a2 = –2
back to replacement

Independent work.

Solve the equations.

2 cosx – 2 = 0

2cos2x – 3cosx +1 = 0

3 sin2x + sinx cosx – 2 cos2x = 0

At the end of independent work, they change jobs and mutually check. The correct answers are projected on the board.

Then they hand it over to an independent expert.

Do it yourself solution

Summing up the lesson.

What type of trigonometric equations did we learn about in class?

Algorithm for solving trigonometric equations of the first and second degree.

Homework: § 20.3 read. No. 361(g), 363(b), additional difficulty No. 380(a).

Crossword.

If you enter the correct words, you will get the name of one of the types of trigonometric equations.

The value of the variable that makes the equation true? (Root)

Unit of measurement for angles? (Radian)

Numerical factor in a product? (Coefficient)

Branch of mathematics that studies trigonometric functions? (Trigonometry)

Which mathematical model necessary for insertion trigonometric functions? (Circle)

Which trigonometric function is even? (Cosine)

What is a true equality called? (Identity)

Equality with a variable? (Equation)

Equations having identical roots? (equivalent)

Set of roots of an equation ? (Solution)

Score sheet

№
n\n

Last name, first name of the teacher

Homework

Presentation

Cognitive activity
studying

Solving equations

Independent
Job

Homework – 12 points (3 equations 4 x 3 = 12 were assigned for homework)

Presentation – 1 point

Student activity – 1 answer – 1 point (4 points maximum)

Solving equations 1 point

Independent work – 4 points

Group rating:

“5” – 22 points or more
“4” – 18 – 21 points
“3” – 12 – 17 points

State budgetary professional educational institution Teeli village, Republic of Tyva

Development of a lesson in mathematics

Lesson topic:

"Homogeneous trigonometric equations"

Teacher: Oorzhak

Ailana Mikhailovna

Lesson topic : “Homogeneous trigonometric equations”(according to the textbook by A.G. Mordkovich)

Group : Master of Plant Growing, 1st year

Lesson type: A lesson in learning new material.

Lesson objectives:

2. Develop logical thinking, the ability to draw conclusions, the ability to evaluate the results of actions taken

3. To instill in students accuracy, a sense of responsibility, and the development of positive motives for learning

Lesson equipment: laptop, projector, screen, cards, posters on trigonometry: meanings of trigonometric functions, basic trigonometry formulas.

Lesson duration: 45 minutes.

Lesson structure:

Progress of the lesson.

1. Organizational moment (1 min)

Check the readiness of students for the lesson, listen to the group on duty.

2. Updating basic knowledge (3 min)

2.1. Checking homework.

Three students solve at the board No. 18.8 (c, d); No. 18.19. The rest of the students do a peer review.

3. Learning new material (13 min)

3.1. Motivation of students.

Students are asked to name equations that they know and can solve (slide No. 1)

1) 3 cos 2 x – 3 cos x = 0;

2) cos (x – 1) = ;

3) 2 sin 2 x + 3 sin x = 0;

4) 6 sin 2 x – 5 cos x + 5 = 0; 1 2

5) sin x cos x + cos²x = 0;

6) tg + 3ctg = 4.

7) 2sin x – 3cos x = 0;

8) sin 2 x + cos 2 x = 0;

9) sin²х – 3sinх cos x+2cos²х = 0.

Students will not be able to name the solution to equations 7-9.

3.2. Explanation of a new topic.

Teacher: Equations that you could not solve are quite common in practice. They are called homogeneous trigonometric equations. Write down the topic of the lesson: “Homogeneous trigonometric equations.” (slide number 2)

On the projector screen definition homogeneous equations. (slide number 3)

Consider a method for solving homogeneous trigonometric equations (slide No. 4, 5)

Analyze the solutions to the examples

Example 1. Solve equation 2sin x – 3cos x = 0; (slide number 6)

This is a homogeneous equation of the first degree. Let's divide both sides of the equation term by cos x , we get:

2tg x – 3 = 0

tg x =

x = arctan + πn , n Z.

Answer: x = arctan + π n, n Z.

Example 2 . Solve sin 2 equation x + cos 2 x = 0; (slide number 7)

This is a homogeneous equation of the first degree. Let's divide both sides of the equation term by cos 2 x , we get:

tg2 x + 1 = 0

tg2 x = - 1

2x = arctan (-1)+ πn, n Z.

2x = - + πn, n Z.

x = - + , n Z.

Answer: x = - + , n Z.

Example 3 . Solve the equation sin²х – 3sinх cos x+2cos²х = 0. (slide number 8)

Each term in the equation has the same degree. This is a homogeneous equation of the second degree. Let's divide both sides of the equation term by term by cos 2 x ≠ 0, we get:

tg 2 x-3tg x+2 = 0. Let's introduce a new variable z = tan x, we get

z 2 – 3z + 2 =0

z 1 = 1, z 2 = 2

this means either tg x = 1 or tg x = 2

4. Consolidation of the studied material (10 min)

The teacher analyzes in detail examples with weak students on the board, strong students solve independently in their notebooks.

5. Differentiated independent work (15 min)

The teacher issues cards with tasks of three levels: basic (A), intermediate (B), advanced (C). Students themselves choose which level of examples they will solve.

6. Summing up. Reflection educational activities in class (2 min)

Answer the questions:

What types of trigonometric equations have we learned?

How to solve a homogeneous equation of the first degree?

How to solve a homogeneous equation of the second degree?

I found out...

I learned...

Mark good job in the lesson of individual students, give grades.

7. Homework. (1 min)

Inform students of their homework and give brief instructions on how to complete it.

No. 18.12 (c, d), No. 18.24 (c, d), No. 18.27 (a)

Used literature:

Slide 2

"Homogeneous trigonometric equations"

1. An equation of the form a sin x + b cos x = 0, where a ≠0, b ≠0 is called a homogeneous trigonometric equation of the first degree. 2. An equation of the form a sin 2 x + b sin x cos x + c cos 2 x = 0, where a ≠0, b ≠0, c ≠0 is called a homogeneous trigonometric equation of the second degree. Definition:

I degree a sinx + b cosx = 0, (a,b ≠ 0). Let's divide both sides of the equation term by term by cosx ≠ 0. We get: a tanx + b = 0 tgx = -b /a the simplest trigonometric equation When dividing a homogeneous equation a sinx + b cosx = 0 by cos x ≠ 0, the roots of this equation are not lost. Method for solving homogeneous trigonometric equations

a sin²x + b sinx cosx + c cos²x = 0. 1) if a ≠ 0, divide both sides of the equation term by cos ² x ≠0 We obtain: a tan ² x + b tgx + c = 0, solve by introducing a new variable z = tgx 2) if a = 0, then we get: b sinx cosx + c cos ² x = 0, solve by factorization method / When dividing the homogeneous equation a sin ² x + b sinx cosx + c cos ² x = 0 by cos 2 x ≠ 0 the roots of this equation are not lost. II degree

This is a homogeneous equation of the first degree. Let's divide both sides of the equation term by term by cos x, we get: Example 1. Solve the equation 2 sin x – 3 cos x = 0

This is a homogeneous equation of the first degree. Let's divide both sides of the equation term by cos 2 x, we get: Example 2. Solve the equation sin 2 x + cos 2 x = 0

Each term in the equation has the same degree. This is a homogeneous equation of the second degree. Let's divide both sides of the equation term by term with os 2 x ≠ 0, we get: Example 3. Solve the equation sin ² x – 3 sin x cos x+2 cos ² x = 0

Answer the questions: - What types of trigonometric equations have we studied? -How to solve a homogeneous equation of the first degree? - How to solve a homogeneous equation of the second degree? Summing up

I learned... - I learned... Reflection

No. 18.12 (c, d), No. 18.24 (c, d), No. 18.27 (a) Homework.

Thanks for the lesson! Well done!

Preview:

Self-analysis of a mathematics lesson by teacher Oorzhak A.M.

Group : Master of Plant Growing, 1st year.

Lesson topic : Homogeneous trigonometric equations.

Lesson type : A lesson in learning new material.

Lesson objectives:

1. To develop students’ skills in solving homogeneous trigonometric equations, to consider methods for solving homogeneous equations of basic and higher level complexity.

2. Develop logical thinking, the ability to draw conclusions, and the ability to evaluate the results of actions performed.

3. To instill in students accuracy, a sense of responsibility, and the development of positive motives for learning.

The lesson was conducted according to thematic planning. The topic of the lesson reflects the theoretical and practical parts of the lesson and is understandable to students. All stages of the lesson were aimed at achieving these goals, taking into account the characteristics of the group.

Lesson structure.

1. The organizational moment included the preliminary organization of the group, the mobilizing beginning of the lesson, the creation of psychological comfort and the preparation of students for the active and conscious assimilation of new material. The preparation of the group and each student was checked visually by me. Didactic task of the stage: Ppositive attitude towards the lesson.

2. The next stage is updating the students’ basic knowledge. The main task of this stage is: restoration in the students’ memory of the knowledge necessary for learning new material. The updating was carried out in the form of checking homework at the board.

3. (Main stage of the lesson) Formation of new knowledge. At this stage, the following didactic tasks were implemented: Ensuring perception, comprehension and primary memorization of knowledge and methods of action, connections and relationships in the object of study.

This was facilitated by: the creation of a problem situation, the method of conversation in combination with the use of ICT. An indicator of the effectiveness of students’ assimilation of new knowledge is the correctness of answers, independent work, active participation students at work.

4.The next stage is the primary consolidation of the material. The purpose of which is to establish feedback to obtain information about the degree of understanding of the new material, completeness, correctness of its assimilation and for timely correction of detected errors. For this I used: solving simple homogeneous trigonometric equations. Here, tasks from the textbook were used that correspond to the required learning outcomes. The initial consolidation of the material was carried out in an atmosphere of goodwill and cooperation. At this stage, I worked with weak students, the rest decided on their own, followed by self-testing from the board.

5. Next moment lesson was the primary control of knowledge. Didactic task of the stage: Identifying the quality and level of mastery of knowledge and methods of action, ensuring their correction. Here, she implemented a differentiated approach to learning and offered the children a choice of tasks at three levels: basic (A), intermediate (B), and advanced (C). I made a round and noted the students who had chosen basic level. These students performed the work under the supervision of the teacher.

6. At the next stage - summing up, the tasks of analyzing and assessing the success of achieving the goal were solved. Summing up the lesson, I simultaneously reflected on the learning activity. Students learned ways to solve homogeneous trigonometric equations. Grades were given.

7. Final stage- homework assignment. Didactic task: Ensuring students understand the content and methods of completing homework. Gave brief instructions on how to do homework.

During the lesson, I was able to realize teaching, developmental and educational goals. I think that this was facilitated by the fact that from the first minutes of the lesson the children showed activity. They were ready to accept a new topic. The atmosphere in the group was psychologically favorable.




Nonlinear equations with two unknowns

Definition 1. Let A be some set of pairs of numbers (x; y) . They say that the set A is given numeric function z from two variables x and y , if a rule is specified with the help of which each pair of numbers from set A is associated with a certain number.

Specifying a numerical function z of two variables x and y is often denote So:

Where f (x , y) – any function other than a function

f (x , y) = ax+by+c ,

where a, b, c are given numbers.

Definition 3. Solving equation (2) call a pair of numbers ( x; y) , for which formula (2) is a true equality.

Example 1. Solve the equation

Since the square of any number is non-negative, it follows from formula (4) that the unknowns x and y satisfy the system of equations



the solution to which is a pair of numbers (6; 3).

Answer: (6; 3)

Example 2. Solve the equation

Therefore, the solution to equation (6) is infinite set pairs of numbers kind

(1 + y ; y) ,

where y is any number.

linear

Definition 4. Solving a system of equations



call a pair of numbers ( x; y) , when substituting them into each of the equations of this system, the correct equality is obtained.

Systems of two equations, one of which is linear, have the form



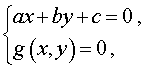
g(x , y)

Example 4. Solve system of equations

Solution . Let us express the unknown y from the first equation of system (7) through the unknown x and substitute the resulting expression into the second equation of the system:





Solving the equation

x 1 = - 1 , x 2 = 9 .

Hence,

y 1 = 8 - x 1 = 9 ,
y 2 = 8 - x 2 = - 1 .





Systems of two equations, one of which is homogeneous

Systems of two equations, one of which is homogeneous, have the form



where a, b, c are given numbers, and g(x , y) – function of two variables x and y.

Example 6. Solve system of equations

Solution . Let's solve the homogeneous equation

3x 2 + 2xy - y 2 = 0 ,

3x 2 + 17xy + 10y 2 = 0 ,

treating it as a quadratic equation with respect to the unknown x:

.

In case x = - 5y, from the second equation of system (11) we obtain the equation

5y 2 = - 20 ,

which has no roots.

In case

from the second equation of system (11) we obtain the equation

,

whose roots are numbers y 1 = 3 , y 2 = - 3 . Finding for each of these values ​​y the corresponding value x, we obtain two solutions to the system: (- 2 ; 3) , (2 ; - 3) .

Answer: (- 2 ; 3) , (2 ; - 3)

Examples of solving systems of equations of other types

Example 8. Solve a system of equations (MIPT)

Solution . Let us introduce new unknowns u and v, which are expressed through x and y according to the formulas:

In order to rewrite system (12) in terms of new unknowns, we first express the unknowns x and y in terms of u and v. From system (13) it follows that

Let us solve the linear system (14) by eliminating the variable x from the second equation of this system. For this purpose, we perform the following transformations on system (14):

• We will leave the first equation of the system unchanged;
• from the second equation we subtract the first equation and replace the second equation of the system with the resulting difference.

As a result, system (14) is transformed into an equivalent system



from which we find

Using formulas (13) and (15), we rewrite the original system (12) in the form

The first equation of system (16) is linear, so we can express from it the unknown u through the unknown v and substitute this expression into the second equation of the system.

With this video lesson, students will be able to study the topic of homogeneous trigonometric equations.

Let's give definitions:

1) a homogeneous trigonometric equation of the first degree looks like a sin x + b cos x = 0;

2) a homogeneous trigonometric equation of the second degree looks like a sin 2 x + b sin x cos x + c cos 2 x = 0.

Consider the equation a sin x + b cos x = 0. If a is equal to zero, then the equation will look like b cos x = 0; if b is equal to zero, then the equation will look like a sin x = 0. These are the equations that we called the simplest and were solved earlier in previous topics.

Now consider the option when a and b are not equal to zero. By dividing the parts of the equation by the cosine x, we carry out the transformation. We get a tg x + b = 0, then tg x will be equal to - b/a.

From the above it follows that the equation a sin mx + b cos mx = 0 is a homogeneous trigonometric equation of degree I. To solve an equation, divide its parts by cos mx.

Let's look at example 1. Solve 7 sin (x/2) - 5 cos (x/2) = 0. First, divide the parts of the equation by cosine (x/2). Knowing that sine divided by cosine is tangent, we get 7 tan (x/2) - 5 = 0. Transforming the expression, we find that the value of tan (x/2) is equal to 5/7. The solution to this equation has the form x = arctan a + πn, in our case x = 2 arctan (5/7) + 2πn.

Consider the equation a sin 2 x + b sin x cos x + c cos 2 x = 0:

1) at a equal to zero the equation will look like b sin x cos x + c cos 2 x = 0. Transforming, we obtain the expression cos x (b sin x + c cos x) = 0 and proceed to solving the two equations. After dividing the parts of the equation by the cosine x, we get b tg x + c = 0, which means tg x = - c/b. Knowing that x = arctan a + πn, then the solution in this case will be x = arctan (- с/b) + πn.

2) if a is not equal to zero, then by dividing the parts of the equation by the cosine squared, we obtain an equation containing a tangent, which will be quadratic. This equation can be solved by introducing a new variable.

3) when c is equal to zero, the equation will take the form a sin 2 x + b sin x cos x = 0. This equation can be solved if we take the sine of x out of brackets.

1. see if the equation contains a sin 2 x;

2. If the equation contains the term a sin 2 x, then the equation can be solved by dividing both sides by the squared cosine and then introducing a new variable.

3. If the equation does not contain a sin 2 x, then the equation can be solved by taking cosx out of brackets.

Let's consider example 2. Let's take the cosine out of brackets and get two equations. The root of the first equation is x = π/2 + πn. To solve the second equation, we divide the parts of this equation by the cosine x, and by transformation we obtain x = π/3 + πn. Answer: x = π/2 + πn and x = π/3 + πn.

Let's solve example 3, an equation of the form 3 sin 2 2x - 2 sin 2x cos 2x + 3 cos 2 2x = 2 and find its roots, which belong to the segment from - π to π. Because This equation is inhomogeneous, it is necessary to bring it to a homogeneous form. Using the formula sin 2 x + cos 2 x = 1, we get the equation sin 2 2x - 2 sin 2x cos 2x + cos 2 2x = 0. Dividing all parts of the equation by cos 2 x, we get tg 2 2x + 2tg 2x + 1 = 0 . Using the input of a new variable z = tan 2x, we solve the equation whose root is z = 1. Then tan 2x = 1, which implies that x = π/8 + (πn)/2. Because according to the conditions of the problem, you need to find the roots that belong to the segment from - π to π, the solution will have the form - π< x <π. Подставляя найденное значение x в данное выражение и преобразовывая его, получим - 2,25 < n < 1,75. Т.к. n - это целые числа, то решению уравнения удовлетворяют значения n: - 2; - 1; 0; 1. При этих значениях n получим корни решения исходного уравнения: x = (- 7π)/8, x = (- 3π)/8, x =π/8, x = 5π/8.

TEXT DECODING:

Homogeneous trigonometric equations

Today we will look at how “Homogeneous trigonometric equations” are solved. These are equations of a special type.

Let's get acquainted with the definition.

Equation of the form and sin x+bcosx = 0 (and sine x plus be cosine x is equal to zero) is called a homogeneous trigonometric equation of the first degree;

equation of the form and sin 2 x+bsin xcosx+scos 2 x= 0 (and sine square x plus be sine x cosine x plus se cosine square x equals zero) is called a homogeneous trigonometric equation of the second degree.

If a=0, then the equation takes the form bcosx = 0.

If b = 0 , then we get and sin x= 0.

These equations are elementary trigonometric, and we discussed their solution in our previous topics

Let's consider the case when both coefficients are not equal to zero. Let's divide both sides of the equation Asinx+ bcosx = 0 member by member cosx.

We can do this since the cosine of x is non-zero. After all, if cosx = 0 , then the equation Asinx+ bcosx = 0 will take the form Asinx = 0 , A≠ 0, therefore sinx = 0 . Which is impossible, because according to the basic trigonometric identity sin 2 x+cos 2 x=1 .

Dividing both sides of the equation Asinx+ bcosx = 0 member by member cosx, we get: + =0

Let's carry out the transformations:

1. Since = tg x, then =and tg x

2 reduce by cosx, Then

Thus we get the following expression and tg x + b =0.



Let's carry out the transformation:

1.move b to the right side of the expression with the opposite sign

and tg x =- b

2. Let's get rid of the multiplier and dividing both sides of the equation by a

tan x= -.

Conclusion: Equation of the form a sinmx+bcosmx = 0 (and sine em x plus be cosine em x equals zero) is also called a homogeneous trigonometric equation of the first degree. To solve it, divide both sides by cosmx.

EXAMPLE 1. Solve the equation 7 sin - 5 cos = 0 (seven sine x over two minus five cosine x over two equals zero)

Solution. Dividing both sides of the equation term by cos, we get

1. = 7 tan (since the ratio of sine to cosine is a tangent, then seven sine x by two divided by cosine x by two is equal to 7 tan x by two)

2. -5 = -5 (with cos abbreviation)

This way we got the equation

7tg - 5 = 0, Let's transform the expression, move minus five to the right side, changing the sign.

We have reduced the equation to the form tg t = a, where t=, a =. And since this equation has a solution for any value A and these solutions have the form

x = arctan a + πn, then the solution to our equation will have the form:

Arctg + πn, find x

x=2 arctan + 2πn.

Answer: x=2 arctan + 2πn.

Let us move on to the homogeneous trigonometric equation of the second degree

Asin 2 x+b sin x cos x +Withcos 2 x= 0.

Let's consider several cases.

I. If a=0, then the equation takes the form bsinxcosx+scos 2 x= 0.

When solving e Then we use the method of factorization of the equations. We'll take it out cosx beyond the bracket and we get: cosx(bsinx+scosx)= 0 . Where cosx= 0 or

b sin x +Withcos x= 0. And we already know how to solve these equations.



Let's divide both sides of the equation term by cosх, we get

1 (since the ratio of sine to cosine is a tangent).

Thus we get the equation: b tg x+c=0

We have reduced the equation to the form tg t = a, where t= x, a =. And since this equation has a solution for any value A and these solutions have the form

x = arctan a + πn, then the solution to our equation will be:

x = arctan + πn, .

II. If a≠0, then we divide both sides of the equation term by term into cos 2 x.

(Arguing in a similar way, as in the case of a homogeneous trigonometric equation of the first degree, cosine x cannot go to zero).

III. If c=0, then the equation takes the form Asin 2 x+ bsinxcosx= 0. This equation can be solved by factorization method (we take out sinx beyond the bracket).

This means that when solving the equation Asin 2 x+ bsinxcosx+scos 2 x= 0 you can follow the algorithm:

EXAMPLE 2. Solve the equation sinxcosx - cos 2 x= 0 (sine x times cosine x minus root of three times cosine squared x equals zero).

Solution. Let's factorize it (put cosx out of brackets). We get

cos x(sin x - cos x)= 0, i.e. cos x=0 or sin x - cos x= 0.

Answer: x =+ πn, x= + πn.

EXAMPLE 3. Solve the equation 3sin 2 2x - 2 sin2xcos2 x +3cos 2 2x= 2 (three sine squared two X minus twice the product of sine two X times cosine two X plus three cosine squared two X) and find its roots belonging to the interval (- π;

Solution. This equation is not homogeneous, so let's make some transformations. We replace the number 2 contained on the right side of the equation with the product 2 1

Since by the main trigonometric identity sin 2 x + cos 2 x =1, then

2 ∙ 1= 2 ∙ (sin 2 x + cos 2 x) = opening the brackets we get: 2 sin 2 x + 2 cos 2 x.

2 ∙ 1= 2 ∙ (sin 2 x + cos 2 x) =2 sin 2 x + 2 cos 2 x

This means that the equation 3sin 2 2x - 2 sin2xcos2 x +3cos 2 2x= 2 will take the form:

3sin 2 2x - 2 sin 2x cos2 x +3cos 2 2x = 2 sin 2 x + 2 cos 2 x.

3sin 2 2x - 2 sin 2x cos2 x +3cos 2 2x - 2 sin 2 x - 2 cos 2 x=0,

sin 2 2x - 2 sin 2x cos2 x +cos 2 2x =0.

We obtained a homogeneous trigonometric equation of the second degree. Let's apply the method of term-by-term division by cos 2 2x:

tg 2 2x - 2tg 2x + 1 = 0.

Let's introduce a new variable z= tan2x.

We have z 2 - 2 z + 1 = 0. This is quadratic equation. Noticing the abbreviated multiplication formula on the left side - the square of the difference (), we obtain (z - 1) 2 = 0, i.e. z = 1. Let's return to the reverse substitution:



We have reduced the equation to the form tg t = a, where t= 2x, a =1. And since this equation has a solution for any value A and these solutions have the form

x = arctan x a + πn, then the solution to our equation will be:

2х= arctan1 + πn,

x = + , (x is equal to the sum of pi times eight and pi en times two).

All we have to do is find values ​​of x that are contained in the interval

(- π; π), i.e. satisfy the double inequality - π x π. Because

x= + , then - π + π. Divide all parts of this inequality by π and multiply by 8, we get

move one to the right and to the left, changing the sign to minus one

divide by four we get,

For convenience, we separate the whole parts in fractions

-

This inequality is satisfied by the following integer n: -2, -1, 0, 1

Maintaining your privacy is important to us. For this reason, we have developed a Privacy Policy that describes how we use and store your information. Please review our privacy practices and let us know if you have any questions.

Collection and use of personal information

Personal information refers to data that can be used to identify or contact a specific person.

You may be asked to provide your personal information at any time when you contact us.

Below are some examples of the types of personal information we may collect and how we may use such information.

What personal information do we collect:

• When you submit an application on the site, we may collect various information, including your name, phone number, email address, etc.

How we use your personal information:

• The personal information we collect allows us to contact you with unique offers, promotions and other events and upcoming events.
• From time to time, we may use your personal information to send important notices and communications.
• We may also use personal information for internal purposes, such as conducting audits, data analysis and various research in order to improve the services we provide and provide you with recommendations regarding our services.
• If you participate in a prize draw, contest or similar promotion, we may use the information you provide to administer such programs.

Disclosure of information to third parties

We do not disclose the information received from you to third parties.

Exceptions:

• If necessary - in accordance with the law, judicial procedure, in legal proceedings, and/or on the basis of public requests or requests from government authorities in the territory of the Russian Federation - to disclose your personal information. We may also disclose information about you if we determine that such disclosure is necessary or appropriate for security, law enforcement, or other public importance purposes.
• In the event of a reorganization, merger, or sale, we may transfer the personal information we collect to the applicable successor third party.

Protection of personal information

We take precautions - including administrative, technical and physical - to protect your personal information from loss, theft, and misuse, as well as unauthorized access, disclosure, alteration and destruction.

Respecting your privacy at the company level

To ensure that your personal information is secure, we communicate privacy and security standards to our employees and strictly enforce privacy practices.